Ultimate Texas Hold 'Em

Last Update: Jan. 29, 2012

Introduction

Ultimate Texas Hold'em is a poker-based casino game in which the player may make one raise at any time during the course of the hand. The earlier the raise is made the higher it may be. Unlike other poker-based games, raises made after the ante still have action, even if the dealer doesn't open. This game was invented by Roger Snow of Shuffle Master .

Rules

1. The game is played with a single ordinary 52-card deck.
2. The player must make an equal bet on both the Ante and Blind, and can also make an optional Trips bet.
3. Two cards are dealt face down to the player and dealer. The player may look at his own cards.
4. The player can check or make a Play bet equal to three or four times the Ante.
5. The dealer turns over three community cards.
6. If the player previously checked, then he may make a Play bet equal to two times his Ante. If the player already made a Play bet, then he may not bet further.
7. Two final community cards are turned over.
8. If the player previously checked twice, then he must either make a Play bet equal to exactly his Ante, or fold, losing both his Ante and Blind bets. If the player already raised he may not bet further.
9. The player and dealer will both make the best possible hand using any combination of their own two cards and the five community cards.
10. The dealer will need at least a pair to open.
11. The following table shows how the Blind, Ante, and Play bets are scored, according to who wins, and whether the dealer opens.

    Scoring Rules
    Winner	Dealer Opens	Blind	Ante	Play
    Player	Yes	Win	Win	Win
    Player	No	Win	Push	Win
    Dealer	Yes	Lose	Lose	Lose
    Delaer	No	Lose	Push	Lose
    Tie	Yes or No	Push	Push	Push

12. Winning Blind and Play bets pay 1 to 1. Winning Blind bets pay according to the following pay table.

    Blind Bet Pay Table
    Player Hand	Pays
    Royal flush	500 to 1
    Straight flush	50 to 1
    Four of a kind	10 to 1
    Full house	3 to 1
    Flush	3 to 2
    Straight	1 to 1
    All other	Push

13. The Trips bet will pay according to the poker value of the player's hand regardless of the value of the dealer's hand, according to one of the Trip bet pay tables below.

Analysis

There are 52 possible outcomes of each hand. The table below shows the probability of each and the contribution to the total return, assuming optimal strategy. A 4X raise is referred to as a "large raise," a 2X raise as "medium," and 1x as "small."

The lower right cell shows a house edge of 2.185% per ante bet. What this means, for example, is if you bet $1 and both the Ante and Blind initially, then you can expect to lose 2.185 cents on average. However for comparison to other games I believe the Element of Risk is more appropriate to look at. The average total amount bet by the end of the hand is 4.152252 times the ante bet. So the element of risk would be 2.185%/4.152252 = 0.526%.

Large bettors should be wary of maximum payouts. If your ante bet is more than 1/500 of the maximum payout, then you will get shortchanged on a royal flush. For every 100 the effective payout on a royal goes down, the house edge will go up by 0.308%. In other words, the increase in the house edge will be [500-(MP/500)]*0.0000308, where MP is the maximum payout.

The next table shows the average wager and return from each bet.

The next table shows the probability of a large, medium, or small raise, or fold for each initial 2-card hand, as well as the expected value.

Strategy

Use my Ultimate Texas Hold 'em Calculator to get the strategy for a specific situation.

The following table shows when to make a 4X raise. The player should never make a 3X raise.

The strategy for the next two decision points is rather long and complicated. I indicate what it is in my Ultimate Texas Hold 'Em advanced strategy.

If my advanced strategy is too complicated, it may help a little to know that when at the medium raise decision point the player will make the 2x raise 34.2% of the time. When at the small raise decision point the player will make the 1x raise 53.2% of the time. There is also a strategy card at discountgambling.net.

Calculator

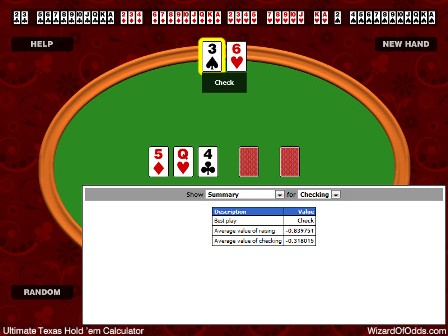
I'm proud to present my Ultimate Texas Hold 'Em calculator. Put in any cards after the flop, river, or turn, and it will tell you the correct play and expected value.

Trips Bet

Shufflemaster literature mentions the following four possible pay tables on the Trips bet.

Pay table #2 seen at the Wynn.

Pay table #3 seen at the Mirage.

Pay table #4 seen at Shufflemaster TableMax units.

Progressive Side Bet — Type 1

Some casinos have a $1 progressive side bet, that pays based on the player's hole cards and five community cards. I saw it on Aug. 17, 2010 at Harrah's in Las Vegas. At the time, the jackpot was $59,327. If the player flops a royal flush then he wins the jackpot. If the player gets a royal flush, using at least one hole card, then he wins 5% of the jackpot. The following return table shows the probability and return for each hand. All pays are on a "for one" basis, meaning the player never gets his original bet back, even if he wins.

The return for at any given time is 50.19% plus 3.00% for each $10,000 in the meter. For exactly zero house edge, the meter would need to be $165,959.74. I'm told the meter is seeded at $5,000, and 27% of money bet goes towards the meter. Fixed wins are not deducted from the meter. That would make the overall return 77.96%.

Progressive Side Bet — Type 2

I have a report that the Firekeeper's casino in Michigan has a $1 progressive jackpot based on the flop and the player's two hole cards. I hear the jackpot seed is $10,000. I don't know the contribution rate. The win for a royal flush is 100% of the jackpot, and 10% for a straight flush. The following return table shows the probability and return for each hand. All pays are on a "for one" basis, meaning the player never gets his original bet back, even if he wins.

For any given jackpot amount, the return is 53.057% + 2.924% for every $10,000 in the jackpot meter. The breakeven point, where there would be zero house edge is at a mater of $160,530.53.

I have an unconfirmed report that the "Michigan Progressive" offers envy bonuses of $300 if another player has a straight flush, and $1,000 for a royal flush. If true, this would add 0.57% to the return for each additional player.

Methodology

This was a difficult game to analyze and required 40 days of computer time to cycle through all the combinations. As with most other poker based games, my analysis was combinatorial in C++, looping through all possible combinations of cards by brute force.

Shufflemaster literature states the element of risk to 0.8%. This figure is based on the analysis of Elliot Fromme. As shown above, my element of risk is slightly lower at 0.526%. So I respectfully disagree with Mr. Fromme.

External Links

Here's a link to Shufflemaster's Ultimate Texas Hold'em page.

Discountgambling.net has an outstanding analysis of this game, including strategy for the second and third decision points.

Game demo , which teaches optimal strategy, by the creator of discountgambling.net.