Video Poker - Deuces Wild
I looked over your expected payouts for the various deuces wild pay schedules, but I did not find the particular schedule I was looking for. Could you tell me the expected payout for a deuces wild with the following schedule:
Royal flush - 840
Four deuces - 200
Wild Royal - 20
Five of a kind - 12
Straight flush - 9
Four of a kind - 5
Full house - 3
Flush - 2
Straight - 2
Three of a kind - 1
I would do this myself, but I am unable to use the necessary software, as I am not a windows user.
The return is 99.0251%.
I noticed that on your full pay Deuces Wild strategy table that one pair (no deuce) is higher on the list that two pair. Does this mean that if I am dealt two pair, that I should always discard three cards and keep only one of the pairs? If so, how do I decide which pair to keep?
Yes, you should only keep one of the two pairs. The only exception to this two pair rule is if you also had three to a royal flush. It does not matter which pair you keep. This is one of the few situations among all games of skill where the optimal strategy player has some free will. Personally I always keep the higher pair, just so I don't slow myself down trying to decide.
How should I play the following hands in deuces wild video poker if both a flush and four of a kind pay 4 to 1? (1) two pair, (2) deuce and two suited high cards
I'm going to assume the rest of the pay table is the same as full pay deuces wild. In this case (1) keep the two pair, (2) keep the deuce and both high cards.
Are there any Internet casinos with looses dueces wild and which casino has the best payouts for dueces wild. Thank you.
Atlantic Interbet has full pay deuces wild (return of 100.77%). I think their highest coinage is 50 cents in that game.
I was running your deuces wild video poker program. I had the following cards. QJ8 of spades T7 of diamonds. I opted for the 4 card inside straight draw and kept the QJT8. The advice thing came back and recommended that I keep the JT87 and discard the Q. This looks like one of those situations where there are two plays with the same EV (similar to the case where you have two pair and need to keep just one pair).
You’re right. There are two ways you can go for an inside straight, both of which have the same expected value. Sorry my program scolded you, I should correct that.
This is an excellent site very interesting and informative. When going through the list of plays in deuces wild video poker I didn’t understand what is meant by a penalty card. Would you please explain.
Thanks for the compliment. I just rewrote my explanation of penalty cards. In my opinion beginning players should not use a strategy that considers penalty cards. However for those who must play near perfectly here is my explanation, "A penalty card is a potentially useful discarded card. For example if the player had 3 to a royal and 4 to a flush the correct play is to keep three to the royal, discarding the fourth suited card. The discarded suited card would be called a flush penalty card because it could have been used to complete a flush. By discarding it the players odds of forming a flush are "penalized." Sometimes penalty cards can affect borderline plays. For example if the player had a suited 10 and king, with no other cards of that suit, nor a 9, jack, queen, or ace, then the player should keep the two to a royal flush. However this is only marginally better than discarding everything. If the player had just one suited card, or any card that could be used to complete a straight, then the odds of forming a flush or straight would be depressed, lowering the overall expected return below that of discarding everything. So in that situation the player should be mindful of the effect of penalty cards."
Went to play deuces wild video poker. There was quite a variation of payoff odds over many machines. None of the payoff odds matched the ones that you have analyzed as having an edge for the player. Is this because the casinos saw your winning strategy and, as a result, changed the odds to make it less favorable to the player? If so thanks a lot Wizard!
I doubt I’m directly responsible but it could be argued that gambling experts like me (Bob Dancer in particular) are what ruined video poker. However if it weren’t for the experts teaching proper strategy then only the experts would know how to play properly.
I have something rather interesting to calculate. I was playing Deuces Wild Video Poker when I was dealt "Garbage". When I threw all five cards away, I was given 4 deuces on the draw, 1,000 coins! What is the probability for 4-Deuces to appear on the draw after throwing all 5 cards away on the deal? Thanks for your time and keep up the good work on your website! P.S. On the same machine, I switched to Deuces Wild Bonus Poker and was dealt 3 wilds w/4&5 of diamonds (straight flush), I threw away the 4&5 and hit 4 deuces w/Ace for another 2,000! What a lucky machine! This was at Soboba Casino in Southern California.
No problem. There are combin(47,5)=1533939 ways to arrange 5 cards out of the 47 left in the deck. 43 of those will result in four deuces (the 5th card has 47-4=43 possibilities). So the probability of getting four deuces on the draw is 43/1533939 = 1/35673 = 0.000028032. The probability of drawing a fourth deuce after keeping 3 is (47-1)/combin(47,2) = 46/1081 = 0.0426. In my video poker appendix 5 you can see how the probability distribution in deuces wild of the number of cards drawn to any given hand. For example 2.62% of all four deuces will be obtained by getting all four on the draw. The probability of this happening on any given hand is 0.000005. For more information on the combin function visit my section on probabilities in poker.
Playing deuces wild if I hold three deuces what is my probability of getting four deuces on the draw? What if I hold two deuces?
If you hold three deuces there are 46 ways you can get the other deuce and another card. There are combin(47,2)=1081 to choose two cards out of 47 left in the deck. So the probability of getting four deuces on the draw with three held is 46/1081 = 4.26% = 1 in 23.5. If you hold two deuces there are 45 ways to get two more deuces plus another card. There are combin(47,3)=16215 ways to choose 3 cards out of 47. So the probability of getting four deuces on the draw after holding two is 45/16215 = 0.28% = 1 in 360.33.
Is it ever a good idea to "double-up" in video poker?
Sure. The double up is one of the few bets with no house edge. For the same reason I advocate the odds in craps I like the double-up in video poker. However if you are playing a game with over 100% return then I recommend against it. Also, if your stomach isn’t strong enough for the extra volatility that comes with the double up feature then you shouldn’t play it. It is also interesting to note that in land casinos cash back does not apply to double-up bets but at Playtech Internet casinos you get 0.1% cash on all bets, including the double-up.
I see that the return on a full pay deuces wild video poker machine is 100.76% with the strategy you have on your website. Obviously, this is with infinite play. My question is how many hands would you have to play to get that return with, let’s say 90% certainty?
p.s. Where can I find full pay full pay deuces wild in Vegas?
There is no number that will give you a 90% probability of attaining or exceeding that percentage. Although the more you play the closer your return will get to 100.76% about half the time it will be above and half the time it will be below. Perhaps a better question is how many hands would I have to play to have a 90% probability of getting to 100.66%?
The standard deviation of full pay deuces wild is 5.08. The standard deviation of the sample mean is 5.08/sqr(n), where n is the number of hands. I’ll skip over the rest of the math to the answer, which is 42,383,720 hands. That is a lot! Suppose your goal is to simply come out ahead with a 90% probability. Then you would only need 733,790 hands. This is still a lot, which just goes to show how volatile your bankroll will be in the short run. The general formula in Excel of your sample return being at least x is with a probability of p is (normsinv(1-p)*5.08/(1.0076-x))^2. In the case of my first example you would put the following in any cell:
=(NORMSINV(1-0.9)*5.08/(1.0076-1.0066))^2
This formula can be used for any game by substituting the correct standard deviation per hand.
I think all the Stations and Fiesta casinos have full pay deuces wild in the quarter coinage only. Green Valley Ranch too. When I moved to Vegas three years ago it was much easier to find.
I think I read somewhere that if someone could come up with a system that had even only 1% player edge, you could easily turn 1000$ into 1000000$. But some video pokers have an 0.77% player edge, why aren’t you turning it into like $770,000 or something? Is it because you can’t bet more than 5$ at a time and it would take WAY too much time? Thx. And oh, I said it before, and I’ll say it again, LOVE your site!!
Thanks! Yes, I said before that if I had a betting system that had just a 1% advantage I could turn $1000 into $1,000,000 by simply grinding out that edge. This would also be possible in video poker but it would take much longer because the 0.77% advantage game (full pay deuces wild) can only be found in the quarter level. Assuming you can play 1000 hands per hour (a speed few can attain) and played perfectly that would result in an average income of $9.63 per hour. To reach $1,000,000 would require working 11.86 years non-stop. $1000 would also be very undercapitalized to play quarter video poker, so the risk of ruin would be quite high. It would be faster to reach the $1,000,000 with the same edge in a table game because the player can bet more.
What is the probability of playing 14,000 hands of deuces wild without getting four deuces?
We can see from my deuces wild section that the probability of four deuces in any one hand is 0.000204. So the probability of not getting four deuces in any one hand is 1-0.000204 = 0.999796. The probability of going 14000 hands without four deuces is 0.99979614000 = 5.75%.
On a full pay deuces wild machine how does a progressive jackpot affect the percent payback. For example on a full pay non-progressive machine the pay out for a Royal Flush no deuces is 4000 coins. This machine has a 100.76 pay out. How is the pay out percentage affected if the Royal Pays 4400 coins?
Assuming no strategy changes for every extra 100 coins in the jackpot the return goes up by 0.044%. So the return with a 4400 coin jackpot would be 100.762% + 4*0.044% = 100.939%.
I was looking at your video poker section and reviewed the "Full Pay" Deuces Wild game with a return of 100.76% using optimum strategy. However, listed below is the "Sevens Wild" game from RTG which I have played at Inet-Bet and Bodog. This pay table is identical to the "Full Pay" Deuces Wild except for the Straight Flush which actually pays 10 for 1 instead of 9 for 1. Shouldn’t this give an expected return greater than 100.76%, and not the 99.11% listed below? Do you know if I am missing something here?
The reason the deuces wild game pays more is because a deuce is not normally as valuable as a seven. This is because there are more ways of making straights and straight flushes around a seven. So making deuces wild is a bigger change than making sevens wild. As I show in my section on Anything’s Wild under the same pay table making deuces wild has a return of 96.76%, while sevens wild is only 94.13%.
In full pay deuces wild the probability of getting a royal flush is about 1 in 40,000. Could it be said the probability in 5-play would be five times easier, or 1 in 8000?
Almost. If more than one royal per deal in 5-play counts as only one sighting then you will have sightings slightly less than 5 times as often. This is because the total number of royals will be five times as much, but sometimes they will be clumped together in the same play, usually when you get a royal on the deal, and thus 5 on the draw.
The following table shows the probability of making a royal in 1-play according to the number of cards to the royal held, assuming full pay optimal strategy.
Royal Flush Probability in 1-Play Video Poker
| Card Held | Probability on deal | Probability on draw | Total probability |
| 0 | 0.19066396 | 0.0000014 | 0.00000027 |
| 1 | 0 | 0.00000561 | 0 |
| 2 | 0.01969711 | 0.00006167 | 0.00000121 |
| 3 | 0.01299751 | 0.00092507 | 0.00001202 |
| 4 | 0.0003309 | 0.0212766 | 0.00000704 |
| 5 | 0.00000154 | 1 | 0.00000154 |
| Total | 0.22369101 | 0 | 0.00002208 |
What this table shows is that 22.37% of the time you will have a possible royal draw. The rest of the time a royal will be impossible, for such reasons as you held a wild card or a pair. The lower right cell shows the overall royal probability is 0.00002208, or 1 in 45282.
The next table shows the same thing but for 5-play, and the probability of at least one royal.
Royal Flush Probability in 5-Play Video Poker
| Card Held | Probability on deal | Probability on draw | Total probability |
| 0 | 0.19066396 | 0.00000698 | 0.00000133 |
| 1 | 0 | 0.00002803 | 0 |
| 2 | 0.01969711 | 0.00030832 | 0.00000607 |
| 3 | 0.01299751 | 0.0046168 | 0.00006001 |
| 4 | 0.0003309 | 0.10195134 | 0.00003374 |
| 5 | 0.00000154 | 1 | 0.00000154 |
| Total | 0.22369101 | 0 | 0.00010268 |
Note the probability of at least one royal is 0.00010268. This is 4.65 as high as the probability for one-play. The reason is the probability of making at least one royal is always less than five times that of 1-play. For example the probaiblity of hitting a royal holding for to the royal is 1/47 in 1-play. However in 5-play the probability of making at least one royal is 1-(1-(1/47))5 = 0.101951341, which is about 4.79 times as high.
Hi, first of all I woulld like to congratulate you for your great site. Very interesting indeed. I have a question regarding your "optimal" strategy in Deuces Wild. In your appendixes about this game you say that you should keep two suited cards or discard everything depending on what you have to discard. In the example you give in appendix 1 the hand is 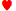 ,
, 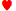 ,
,  ,
,  . In this case everything should be discarded. Now if you get the same hand with an 8d as last card (instead of the 7d),then you should keep the suited Kh and Qh. My question is what difference does it make? Whatever you discard (3c,4d,7
. In this case everything should be discarded. Now if you get the same hand with an 8d as last card (instead of the 7d),then you should keep the suited Kh and Qh. My question is what difference does it make? Whatever you discard (3c,4d,7 or 3c,4
or 3c,4 ,8
,8 ) the odds for getting a royal flush (or straight flush or even anything else) seem the same for me. Since you already have two cards for the Royal Flush why not keep them whatever you have to discard?
) the odds for getting a royal flush (or straight flush or even anything else) seem the same for me. Since you already have two cards for the Royal Flush why not keep them whatever you have to discard?
Thanks. I’ve answered penalty card questions before but because it is an important concept I’ll do it again. You are right that the expected value keeping the king and queen is the same in both hands. However, the value of tossing everything is a little bit more with the 7d than the 8d. To be exact, if you toss everything with the 7 there are 1,606 ways to make a straight, and with the 8d there are only 1,591. The more spread apart your discards are, the harder it will be to make a straight on the draw. This particular case is very borderline. Those 15 extra ways to make a straight by tossing the 7
there are 1,606 ways to make a straight, and with the 8d there are only 1,591. The more spread apart your discards are, the harder it will be to make a straight on the draw. This particular case is very borderline. Those 15 extra ways to make a straight by tossing the 7 pushes the value of tossing everything over that of keeping two to a royal. For the same reason, in a six-deck game of blackjack you double A2 against a 5 but not a 4. The value of doubling is the same in both cases, but the value of hitting is more against the 4, pushing it above the value of doubling.
pushes the value of tossing everything over that of keeping two to a royal. For the same reason, in a six-deck game of blackjack you double A2 against a 5 but not a 4. The value of doubling is the same in both cases, but the value of hitting is more against the 4, pushing it above the value of doubling.
I was playing a triple-play deuces wild machine and was delighted to be dealt four deuces. I kept the deuces and discarded the queen and hit the draw button. Of course I was paid accordingly, but the stranger next to me freaked out saying that I should have held the queen instead of drawing a new card. He cited the fact that malfunctions void all payouts. In future situations, should I really be concerned about such malfunction possibilities?
No. Malfunctions in any video based game are extremely rare. In slots with moving parts they are more likely, but still on the order of one in a million. In video poker, mid-play malfunctions are virtually unheard of. The reason usually given for holding all five cards with four deuces is that otherwise you might hold the wrong cards by pressing the buttons incorrectly. In my opinion the probability of this kind of human error is much greater than the probability of a malfunction.
If the local casino offers me $10 in free play and then requires that I add a dollar to activate it, then how much is the offer worth theoretically? This is an offer I got on a postcard from Sunset Station Casino.
The value of that offer is $10.77, because the Sunset Station has full pay deuces wild, at a return of 100.76% (source: VPfree.com). The money you have to put in first is called "seed money." You don't need to actually play it. When you press "deal" the amount you bet will be deducted from the $10 in free play. If you get something back, it will be added to your real money balance. If you bet through the $10 exactly, you can cash out the real money balance, without ever actually betting the seed money. Personally, I find the seed money requirement annoying. If you are planning to do the free play only, it is an extra nuisance. At the Wynn and Venetian, no seed money is required when collecting free play.
Given that the odds of a Royal in full pay deuces wild is approximately 1 in 49,000, what is the probability of hitting exactly two in 6,000 hands? My wife and I just returned from the Red Rock, where I did this.
The probability of hitting a royal is actually 1 in 45,282 per hand. The probability of hitting exactly two royals in 6,000 hands is combin(6000,2)×(1/45282)2×(45281/45282)5998 = 0.007688177, or 1 in 130.
Say you are playing bonus deuces wild (or any other game where the correct strategy is to hold and draw to only one pair out of two dealt pairs), when playing this game on Spin Poker with 9 patterns, for equal value pairs, does the position of each pair make any difference in terms of which pair should be held and if so, which are the best and worst positions to hold?
For the benefit of other readers, sometimes in deuces wild games the odds favor holding a single pair over a two pair. This is true in full pay deuces wild (100.76%) and any common version of bonus deuces where a full house pays 3. An exact calculation of this would be very tedious and time consuming. However, it is easy to see that on reels 1, 2, 4, and 5, the nine pay-lines run through each position three times. Yet on reel 3, the top and bottom positions are crossed only two times each and the middle position 5 times. It will lower your volatility to hold a pair that includes the middle column. In the 20% of cases where the middle column is the singleton, I would hold a pair if it consists of columns 1 and 5, or 2 and 4, if you can. If that is not possible, then hold a pair in columns 1 and 2, or 4 and 5, if you can. Otherwise, it doesn’t make any difference which pair you hold.
Suppose a casino had a video poker game that was over 100%, but any given player is only allowed to play it until he hits one royal. Should any strategy changes be made?
If you want to be a perfectionist, yes. Let’s look at full pay deuces wild, for example. Normally the return is 1.00762 and a royal hits once every 45282 hands. That makes the expected profit 45282 × (1.00762 - 1) = 345.05 bet units. For a greater overall expected profit, I recommend using a less aggressive royal strategy to increase the total hands played.
In this case, the profit is maximized by following a strategy based on a royal win of 450. That will lower the actual return to 1.007534 and decrease the royal probability to 1 in 46415, resulting in an expected profit of 46415 ×(1.007534-1) = 349.68. The extra 4.6 bet units may not be worth the bother of learning a different strategy.
To find the optimal target royal value, you can use my video poker calculator, and keep lowering the pay for a royal until the overall return gets as close to 1 as possible. At that point, it is like playing for free until you hit the royal, at which point you get a bonus for the royal. In the full pay deuces wild example, the bonus is 800-450=350.
The situation is not entirely hypothetical. Slot managers have been known to prohibit advantage players from playing video poker, and usually such players get the tap on the shoulder shortly after hitting a royal.
Some gambling books say that the correct Kelly bet is advantage/variance. However, you say that is just an approximation and the correct answer is to maximize the expected log of the bankroll after the bet. My question is, how much error is there in the variance approximation?
Advantage/variance is a pretty good approximation. Let’s look at full pay deuces wild, for example. The variance formula says to make a bet of 0.000295 times bankroll. Exact Kelly results in a bet of 0.000345 times bankroll.


