On this page
Keno Explosion
Introduction
Keno Explosion is a keno variant by IGT. It entails placing bombs on three unchosen numbers by the player. If the ball draw matches the number of a bomb, it will explode and if a fragment of the explosion hits a number chosen by the player, it counts as a hit.
Rules
- The player shall pick 2 to 10 numbers from 1 to 80.
- The game shall randomly pick three numbers that were unchosen by the player and mark them with bombs.
- The game shall draw 20 numbers, without replacement, from 1 to 80.
- If any ball drawn by the game matches a number chosen by the player, it shall be counted as a "catch."
- If the ball draw by the game matches one of the three numbers marked by a bomb, then that bomb shall explode. After the explosion, one to three fragments will land on one of the 57 numbers not chosen by the game or marked with a bomb.
- No number may be hit by two or more fragments.
- If the fragment of a bomb lands on a number chosen by the player, it shall also be counted as a "catch."
- The average number of fragments per bomb, based on a small sampling of 27 bombs, is 1.85.
- The player shall be paid according to the number of catches and the posted pay table.
- The pay table below is just an example, which was seen at the Red Rock casino in Las Vegas for a 10¢ bet.
If these rules were unclear, here are screenshots of the rule screens. Click on either image for a larger version.
 |
 |
The following table shows an example pay table. Note that many pays end in 0.5. To keep the total pays in round numbers, the player must bet an even number of coins.
Keno Explosion Pay Table
| Catch | Pick 2 | Pick 3 | Pick 4 | Pick 5 | Pick 6 | Pick 7 | Pick 8 | Pick 9 | Pick 10 |
|---|---|---|---|---|---|---|---|---|---|
| 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 1 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 | 0 |
| 2 | 12 | 2 | 1 | 0 | 0 | 0 | 0 | 0 | 0 |
| 3 | 32.5 | 3.5 | 2 | 1.5 | 0.5 | 0 | 0 | 0 | |
| 4 | 107 | 12.5 | 4 | 1.5 | 1 | 0.5 | 0 | ||
| 5 | 450 | 53 | 13 | 7 | 3 | 2.5 | |||
| 6 | 1000 | 217 | 54 | 23 | 12 | ||||
| 7 | 3750 | 835 | 171 | 77 | |||||
| 8 | 5000 | 2400 | 505 | ||||||
| 9 | 5000 | 2300 | |||||||
| 10 | 5000 |
Example
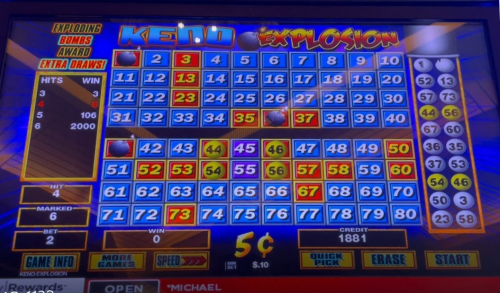
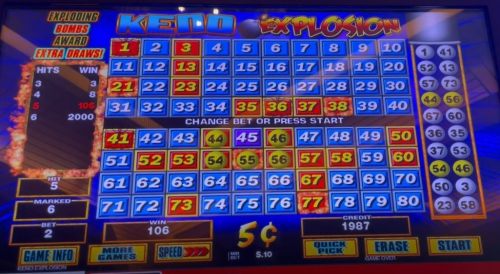
The image below shows a game where I picked six numbers -- 44, 45, 46, 54, 55, and 56. The bombs were placed on 1, 36, and 41. This image was taken after the ball draw, but before the bombs exploded. Note that before the bombs exploded, I caught four of my six numbers, for a win of 8.
The image above was taken after the bombs exploded. Fragments of the bombs hit 21, 38, 55, and 77. You can tell because the background to these numbers are red and also look like they are on fire. Numbers previously occupied by a bomb, 1, 36, and 41 are shown to look broken. One of the fragments hit 55, which was one of the numbers I chose. This increased my number of catches from 4 to 5, which increased my win from 8 to 106.
Are Bombs Gaffed to Explode?
The following table shows the probability of 0 to 3 bombs being caught in the 20 ball draw by the game, assuming natural probabilities of any given bomb having a 20/80 = 25% of matching the ball draw. Note the probability of all three bombs matching the ball draw is 0.013875.
Expected Bomb Explosions
| Bomb Catches |
Combinations | Probability |
|---|---|---|
| 3 | 1140 | 0.013875 |
| 2 | 11400 | 0.138754 |
| 1 | 35400 | 0.430867 |
| 0 | 34220 | 0.416504 |
| Total | 82160 | 1.000000 |
The following table shows the number of bombs that were hit by the draw of the game in a small sampling of 208 games. The right column shows the number of expected games with the given number of bomb catches in 208 games.
Bomb Catches Sampling
| Bomb Catches |
Sample Observations |
Expected |
|---|---|---|
| 3 | 30 | 2.89 |
| 2 | 34 | 28.86 |
| 1 | 25 | 89.62 |
| 0 | 119 | 86.63 |
| Total | 208 | 208.00 |
Note that in 208 games, 30 resulted in all three bombs exploding. If the game followed natural odds, the expected number of game that saw all three bombs explode would be 2.89.
The probability of 30 or more bombs exploding in 208 games is 17.78 standard deviations away from expectations. The probability of 30 or more observations is 1 in 20,840,430,698,432,300,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000.
A chi-squared test on the observations and expectations above has a probability of results that skewed or more of 1 in 12,703,492,578,520,200,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000,000.
It should be noted the first rule screen says, "Explosions and fragments are evaluated after all numbers are drawn." While this is open to interpretation, it suggests to me everything that goes on with the bombs is determined independently and not necessarily by the random draw of balls.
I would like to emphasize that if this game is gaffed, it is gaffed in the player's favor. However, I claim gaffing in either direction is in violation of Nevada Revised Statute 14.040.5, which states, "For gaming devices that are representative of live gambling games, the mathematical probability of a symbol or other element appearing in a game outcome must be equal to the mathematical probability of that symbol or element occurring in the live gambling game."
A random simulation of this game, that assumed natural odds for all aspects, showed a return of 82.49%. As far as I know, IGT, the makers of the game, only allow settings as low as 85%. Other 5¢ keno games on the same machine at the Red Rock were set to an average return of 89.45%. This is further evidence the game is gaffed so the ball draw is more likely to hit bombs than a game that a natural ball draw, where every number was equally likely to be drawn.
Video
In this video I present my evidence and argument for why Keno Explosion does not conform to the natural probabilities of live keno.