Video Poker - General Questions
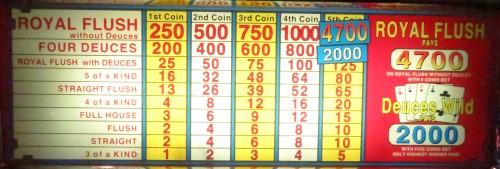
I'm playing an 8/5 bonus machine that pays $1,199 for a royal flush and double for four 8's, in addition to the normal bonus schedule for four 2’s, 3’s, 4’s, and aces. My slot card and max coins are required. Is this 100%+?
The $1199 payoff is amusing, just under the $1200 limit for which the casino would be obligated to report the win to the IRS. Winpoker 6 software allows for analysis of custom designed games. In this case, the return is 100.0079%.
Does a video poker game, whether it be jacks or better or any wild version, play like an actual deck of real cards? In other words, the payoff schedule on the front of the machine determines what exact payback this machine has or can this be tinkered with inside with computer chips making the payoff schedule meaningless? I always thought this would be dishonest until I read an article in Strictly Slots magazine that it has and can be done. If this is true you could have two identical video poker machines side by side that have different house edges like. I know casinos can and in regular slot machines. If this is so then all this VP payback percentage on payoff schedules that I have been reading for years in magazines, software and books is useless.
I am very confident that any respectable maker of video poker machines makes them fair and accurate. Is it possible there are dishonest machines or chips out there? Sure. I would be interested to read the article you refer to.
I’m thinking of visiting your advertisers’ web sites just to make sure they keep supporting your ability to give free advice to the gambling public. Your site is great. My question: Is it possible to draw the same card that is discarded in video poker? The Michigan Gaming Control Board has given me a half-answer, stating that all events are determined by a random number generator. Secondly, are the chances of hitting paying hands in VP subject to manipulation by the casino, like slot machines that are also governed by a random number generator?
Thanks for your support. I encourage everyone to click through the banners to help support the site. To answer your question, no, it is not possible to get the same card back. The machine represents a fair deal from a single deck. Once you discard a card you can’t get it back. To the answer the second question, no, the casino can not change the probability of each hand. The video poker machines deal a fair game. How often you get each hand depends on the random draw of the cards and your skill in playing them.
In Mississippi, is the law the same for video poker as in Nevada, that each hand is a new game?
I assume you are asking whether each hand is dealt from a fresh deck, with no memory of past hands. This is how video poker is supposed to be played and I’m sure Mississippi is no exception.
Your internet newspaper column has not been updated since June. Are you OK? I hope so. I have a Black Jack basic strategy card and it is very beneficial. Are there basic strategy cards for electronic poker games? Thanks for your time.
I'm not sure what Internet newspaper column you are referring to. However I'm okay. As a matter of fact you can get my own video poker strategies in a handy dandy strategy card at Custom Strategy Cards. The video poker cards may not be listed yet but I know the proprietor has them so just ask.
Update: The Custom Strategy Cards business no longer exists.
Dear Mr. Wizard, How do minimum payback laws affect video poker machines? Can a casino have a VP machine installed if an ideal strategy is not known? Can really stupid players (ones who would discard a paying pair or even a pat royal) sue a casino if their strategy results in payoffs under x%, as mandated by state law? Finally, out of curiosity, what is the lowest return possible on a VP machine, assuming discarding royal flush, keeping all 5 cards of a garbage hand, etc? Thank you for your valuable time in reading and hopefully responding.
Nevada Gaming Control Board regulation 14.040.1(a) states that gaming devices must return at least 75% assuming optimal player strategy. To answer your second question I modified my video poker program to always make the worst possible play. For example, keeping all five cards on a non-paying hand , and tossing part or all of pat hands. Based on 9/6 Jacks or Better this strategy results in a return of 2.72%, or house edge of 97.28%. Following is the complete return table. Such a player would not be able to sue the casino because it was his fault for playing so badly.
Jacks or Better - Worst Possible Player
| Hand | Payoff | Number | Probability | Return |
|---|---|---|---|---|
| Royal flush | 800 | 48564 | 0.000000 | 0.000002 |
| Straight flush | 50 | 2058000 | 0.000000 | 0.000005 |
| 4 of a kind | 25 | 38040380 | 0.000002 | 0.000048 |
| Full house | 9 | 292922028 | 0.000015 | 0.000132 |
| Flush | 6 | 336550092 | 0.000017 | 0.000101 |
| Straight | 4 | 6239759724 | 0.000313 | 0.001252 |
| 3 of a kind | 3 | 12510891616 | 0.000628 | 0.001883 |
| Two pair | 2 | 34968642984 | 0.001754 | 0.003509 |
| Jacks or better | 1 | 334574728656 | 0.016785 | 0.016785 |
| Nothing | 0 | 19544266875156 | 0.980487 | 0.000000 |
| Total | 19933230517200 | 1.000000 | 0.023717 |
If you had to pick one video poker game to practice on line,(so you knew how to play when in Vegas), what would it be?
Everything you need is right here!
There is my video poker game to play, which will correct you when you make mistakes.
There is my hand analyzer to analyze how to play any hand.
There is my game return calculator to determine the return of any game and pay table.
Finally, there is my strategy maker to create a strategy for any game.
Note: This answer was updated Nov. 13, 2013.
If I put a $100 bill in a 98% return video poker machine and play until I go broke then how much on average will I bet in total?
There is a simple formula for this answer. It the initial investment divided by the house edge. In this case the answer is $100/0.02 = $5000. However due to the volatility of video poker, most of the time the $100 won’t last this long.
Any advice to us folks in Connecticut, with the absolute worst video poker machines in the world? Is there an accepted way to pressure a casino to improve their selection when the market doesn’t necessarily require it?
That is what happens in an environment with almost no competition. Asking the casino for better machines won’t help. If players will play games with lousy pay tables why should they change anything? Your only hope is to write to the lawmakers in Connecticut and ask them to end the two-casino monopoly on gaming in that state and allow others to compete.
If you were playing $50 per play, what would you personally chose between these two games of video poker (assuming both games have the same pay schedules and you bet the maximum of 5 coins for each hand): single-play at $10 or ten-play at $1 per hand? Thanks for your time and consideration.
Mathematically they of course have the same expected return. However I would play the 10-play because the volatility is less and I think it is more fun.
There are a lot of free online video poker games available. However, I am looking for a version I can run without being connected to the Internet. Do you know of a free downloadable version of Jacks or Better that does not use the Internet while running? I’ve found some free download demos, but they still connect to a site when they run even though the game is loading locally. Just a nice basic, realistic (functionally) version would be great. Thanks.
I believe the free Winpoker demo will do that and never expire. The download is available at www.zamzone.com.
What is the coefficient of skewness for video poker?
For the benefit of other readers, the coefficient of skewness (skew) for any random variable is a measure of which direction has the longer tail. A negative skew means the most likely outcomes are on the high side of the distribution, offset by the extremes tending to be on the low side. A positive skew is the opposite, where the most likely outcomes are the low side, but with the extremes tending to be on the high side. The mean is less than the median with a negative skew, and greater with a positive skew. An exact formula can be found at Wikipedia, or lots of statistics books.
Loosely stated, skewness is going to correlate with how often you get a win in a session. In Jacks or Better, for the most part, you are not going to get a winning session over a few hours if you do not hit a royal. You can sit down at Double Double Bonus and be a winner after a few hours more often because of the big quad payouts. Because most people are subject to cognitive biases, the pain from a loss is twice the pleasure from a win. People do not really play Double Double Bonus because they like the variance, they play because they have a greater shot at winning. The following table shows some key statistics for four common video poker games. It is interesting to note that skew is greatest for Jacks or Better.
Key Video Poker Statistics
| Statistic | JoB — 9/6 | BP — 8/5 | DDB — 9/6 | DW — NSUD |
|---|---|---|---|---|
| Return | 0.995439 | 0.99166 | 0.989808 | 0.997283 |
| Variance | 19.514676 | 20.904113 | 41.985037 | 25.780267 |
| Skew | 147.114643 | 134.412152 | 66.495372 | 101.23991 |
| (Excess) Kurtosis | 26,498 | 23,202 | 6,679 | 14,550 |
JoB — 9/6 = Full pay Jacks or Better
BP — 8/5 = Standard pay Bonus Poker
DDB — 9/6 = Standard pay Double Double Bonus Poker
DW — NSUD = "Not so Ugly Ducks" Deuces Wild
How can knowing this actually help the video poker player? I suppose one could say that a game with a large skew has a greater chance of a loss over a session of a few hours. For example, in Jacks or Better, if you don’t hit any royals, the house edge will probably eventually grind your bankroll down. However in a game like Deuces Wild or Double Double Bonus, the second highest wins can pull you out of the hole over a session. In other words, the skew keeps you from winning when you are not hitting royals. Knowing the skew won’t increase your odds, but it is mentally helpful to know what to expect. So, the next time you take a beating in 9/6 Jacks, blame it on the skew.
My thanks to Jeff B. for his help with this question.
I was playing video poker in Atlantic City, and I could have sworn I received the same card I discarded on the draw sometimes. If the machine was putting the discards back in the deck, what would be the effect on the odds?
Cryptologic Internet casinos have such a game, called Bonus Video Poker, where discards are put back in the deck. The 40-20-9-6 Jacks or Better pay table in that game returns 95.2642%, before considering the redraw bonus feature, which I won’t get into here. In conventional video poker, that pay table would return 98.2534%. So, in this example, putting the discards back in the deck costs the player almost 3%. However, I highly doubt that actually happened on a machine in Atlantic City.
This question was raised and discussed in the forum of my companion site Wizard of Vegas.
Which video poker game offers the best chance at a royal?
Let's start by looking at some no-nonsense video poker games. The following table shows the probability of a royal, from highest to lowest. This table does not count wild royals, which pay much less than a natural.
Video Poker Royal Probability
| Game | Probability | Inverse |
|---|---|---|
| 17-7 Joker Poker | 0.0000259892 | 38,478 |
| 8-5 Bonus Poker | 0.0000248551 | 40,233 |
| 9-6 Jacks | 0.0000247583 | 40,391 |
| 9-6 Double Double Bonus | 0.0000245102 | 40,799 |
| 9-6 Bonus Poker Deluxe | 0.0000237661 | 42,077 |
| "Full pay" deuces wild | 0.0000220839 | 45,282 |
| 10-7 Double Bonus | 0.0000208125 | 48,048 |
Surprisingly, Joker Poker is the most likely to get a natural royal. This surprises me because of the extra card, which can't be used to make a natural royal.
Next, let's look at some non-standard video poker games.
In 9-6 Jacks Royal Draw, the odds are 1 in 12,178.
In 7-5 Jacks Second Chance Royal, the odds are 1 in 10,827. This includes "second chance" royals, which pay 200 only.
Finally, I think an honorable mention should be made for Triple Double Bonus, where four aces plus a 2-4 kicker pay the same 800 per coin bet as a royal flush. The odds of either 800 win are 1 in 10,823, based on the 9-7 pay table. Even better is Royal Aces Bonus Poker, which pays 800 for any royal flush or four aces, for a probability of 1 in 3,673 for a win of 800.
However, all things considered, my answer for the game with the most natural royals is Chase the Royal. Based on the 9-6 Jacks pay table, the probability of a royal flush is 1 in 9,084.
Why does the number 19,933,230,517,200 come up so often as the total combinations in video poker?
For the benefit of other readers, here is the return table for 9-6 Jacks or Better.
"9-6" Jacks or Better
| Hand | Payoff | Combinations | Probability | Return |
|---|---|---|---|---|
| Royal flush | 800 | 493512264 | 0.00002476 | 0.01980661 |
| Straight flush | 50 | 2178883296 | 0.00010931 | 0.00546545 |
| Four of a kind | 25 | 47093167764 | 0.00236255 | 0.05906364 |
| Full house | 9 | 229475482596 | 0.01151221 | 0.10360987 |
| Flush | 6 | 219554786160 | 0.01101451 | 0.06608707 |
| Straight | 4 | 223837565784 | 0.01122937 | 0.04491747 |
| Three of a kind | 3 | 1484003070324 | 0.07444870 | 0.22334610 |
| Two pair | 2 | 2576946164148 | 0.12927890 | 0.25855780 |
| Jacks or Better | 1 | 4277372890968 | 0.21458503 | 0.21458503 |
| Nothing | 0 | 10872274993896 | 0.54543467 | 0 |
| Total | 19933230517200 | 1 | 0.99543904 |
Most of my video poker return tables for 52-card games have the same number of combinations of 19933230517200. The question is why?
First, there are combin(52,5) = 2,598,960 ways to choose five cards out of 52.
Second, there are up to combin(47,5) = 1,533,939 combinations on the draw, depending on how many cards the player discards. The following table shows the number of draw combinations in the second column according to the number of discards.
Draw Combinations
| Discard | Combinations | Weight | Product |
|---|---|---|---|
| 0 | 1 | 7,669,695 | 7,669,695 |
| 1 | 47 | 163,185 | 7,669,695 |
| 2 | 1,081 | 7,095 | 7,669,695 |
| 3 | 16,215 | 473 | 7,669,695 |
| 4 | 178,365 | 43 | 7,669,695 |
| 5 | 1,533,939 | 5 | 7,669,695 |
The least common multiple of the numbers in the second column is 7,669,695. This number can be expressed as 5×combin(47,5). To keep the total number of combinations the same for every hand on the deal I weight the draw combinations so that the total combinations on the draw is 7,669,695.
So, 19,933,230,517,200 = combin(52,5)×combin(47,5)×5. Some of my 52-card video poker tables have a smaller number of combinations. This is because sometimes the total number of combinations for each hand in the final return table has a greatest common divisor greater than one. In this case I sometimes divide each total by whatever the greatest common divisor is. My video poker analyzer does this automatically.
This question was raised and discussed at my Wizard of Vegas forum.
Is it true that the D casino took out their 101.60% Loose Deuces game? I recall you saying that was the loosest video poker machine in Vegas. If the rumors are true, which machine is number one now?
Yes, that machine is no longer. As you point out, the previous number two game now bubbles up to the number one spot. That is a 100.92% Double Deuces game at the El Cortez. The full pay table is 940-400-25-16-13-4-3-2-2-1. It can be found on the slot room on the north end of the casino full of old cathode raw machines. Here is a picture of me standing next to it.

In case you're wondering, the difference between Loose Deuces and Double Deuces is the former pays 500 for four deuces and the latter 400.
I know there are some hands in video poker where two plays are equally good as the best play. For example, with a two pair in full pay deuces wild, the player should keep just one pair, and it doesn't matter which one. My question is whether there are any situations where there are three plays that are equally good as the correct play?
Yes! In joker poker, with a garbage hand, as a general rule it is best to keep one of the middle cards that has the best chances of forming a straight or flush on the draw. However, sometimes it is a close call, or even a tie, which is the best card to hold onto.
For example, in the 97.19% pay table for two pair joker poker and a Qh, 10d, 5d, 7c, and 2c on the draw, the best play is a tie between holding only the 10d, 5d, and 7c, with an expected value of 0.240703 for all three. This can be verified using my video poker hand analyzer.
Here are other such plays for the same joker poker pay table:
- QC 10S 5D 7H 2C
- QH 10D 5D 7H 2C
- KH 10D 5D 8C 3C
- KC 10S 5D 8H 3C
- KH 10D 5D 8H 3C
My thanks to Gary Koehler for his help with this question.
Hello. I am writing because I am a big fan of video poker (although I am
not a gambler and play strictly for fun in simulations). I wanted to play
around with some simulations and found your page My Methodology for Video
Poker Analysis immensely helpful. Although it took several tries to
actually wrap my mind around it, I eventually managed to write a little
simulator to auto-play games and can check my results against your Video Poker Hand Analyzer page and get the same answers.
But the methodology page only deals with a standard 52-card deck with no
wild cards. I have tried to expand the algorithm on my own to allow adding
one to four jokers to the deck, but so far the results have been slow and
inaccurate. Part of the problem is that wild cards seem to add a lot of
complexity as my algorithm substitutes 52 possibilities for the wild cards
and furthermore I don't think having duplicate indexes is allowed by the
algorithm. I was wondering if the methodology page could be expanded to
give tips for dealing with wild card situations in a similarly efficient
way that it already deals with the standard deck.
I love the website and want to thank you for the assistance it has provided
to this point, as I don't think I could have completed my project without
it. Have a great day!
Thank you for all the kind words!
Cycling through all 52 ways to use a wild card will definitely be slow, especially if there are multiple wild cards. Here is how I score a hand with wild cards:
- If you have a natural royal flush, then score the hand as such.
- Otherwise, count the number of wild cards in the hand.
- Then score the hand according to this number and the value of the other cards.
For example, suppose you know you have two wild cards. You could use this pseudo-code to score the hand based on the other three cards:
- If the three cards are suited and the lowest one is at least a ten, then you have a wild royal.
- Otherwise, if the three cards are suited and the difference between the highest and smallest is four or less, then you have a straight flush.
- Otherwise, if the three cards are suited, the highest is an ace, and the second highest is a five or less, then you also have a straight flush (A2345).
- Otherwise, if you have a pair, then you have a four of a kind.
- Otherwise, if the three cards are suited, you have a flush.
- Otherwise, if the difference between the highest and smallest is four or less, then you have a straight.
- Otherwise, if the highest is an ace, and the second highest is a five or less, then you also have a straight (A2345).
- Otherwise, you have a pair.
You already have code for no wilds, so you'll have to write separate code for one to either four or five wilds, depending on whether you may wish to analyze Deuces and Joker Wild.
I know of a promotion that pays a bonus after achieving a four of a kind in all 13 ranks. How many hands will this take on average?
Let's look at the gold standard of video poker, 9-6 Jacks or Better to answer your question.
The first step is to modify my calculator to include a line item for all 13 four of a kinds. Here is that modified return table:
Modified Jacks or Better Return Table
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Royal flush | 800 | 493,512,264 | 0.000025 | 0.019807 |
| Straight flush | 50 | 2,178,883,296 | 0.000109 | 0.005465 |
| Four A | 25 | 3,900,253,596 | 0.000196 | 0.004892 |
| Four K | 25 | 3,904,533,816 | 0.000196 | 0.004897 |
| Four Q | 25 | 3,898,370,196 | 0.000196 | 0.004889 |
| Four J | 25 | 3,886,872,684 | 0.000195 | 0.004875 |
| Four 10 | 25 | 3,471,687,732 | 0.000174 | 0.004354 |
| Four 9 | 25 | 3,503,226,684 | 0.000176 | 0.004394 |
| Four 8 | 25 | 3,504,128,652 | 0.000176 | 0.004395 |
| Four 7 | 25 | 3,504,825,252 | 0.000176 | 0.004396 |
| Four 6 | 25 | 3,504,861,888 | 0.000176 | 0.004396 |
| Four 5 | 25 | 3,504,895,944 | 0.000176 | 0.004396 |
| Four 4 | 25 | 3,504,032,676 | 0.000176 | 0.004395 |
| Four 3 | 25 | 3,503,177,148 | 0.000176 | 0.004394 |
| Four 2 | 25 | 3,502,301,496 | 0.000176 | 0.004393 |
| Full house | 9 | 229,475,482,596 | 0.011512 | 0.103610 |
| Flush | 6 | 219,554,786,160 | 0.011015 | 0.066087 |
| Straight | 4 | 223,837,565,784 | 0.011229 | 0.044917 |
| Three of a kind | 3 | 1,484,003,070,324 | 0.074449 | 0.223346 |
| Two pair | 2 | 2,576,946,164,148 | 0.129279 | 0.258558 |
| Jacks or better | 1 | 4,277,372,890,968 | 0.214585 | 0.214585 |
| Nothing | 0 | 10,872,274,993,896 | 0.545435 | 0.000000 |
| Total | 19,933,230,517,200 | 1.000000 | 0.995439 |
The probability of getting any four of a kind is 0.002363.
The next question to be answered is how many four of a kinds will take on average to get all 13 kinds? To answer that question, I created my Expected Trials Calculator. To use it, enter the number of combinations of each four of a kind in the first 13 cells. The calculator will tell you that it will take an expected 41.532646 four of a kinds to get all 13 kinds.
So, the expected number of hands played to get all 13 four of a kinds is 41.341739/0.002363 = 17,580.
What is the cost to not playing all four lines in Multi-Strike poker?
Let's look at 8-5 Bonus Poker as an example. The following table shows the return by number of lines bet.
- 4 lines: 99.375%
- 3 lines: 99.279%
- 2 lines: 99.214%
- 1 line: 99.166%
The next list shows the cost to not playing the maximum lines according to how many lines are played.
- 4 lines: 0.000%
- 3 lines: 0.095%
- 2 lines: 0.160%
- 1 line: 0.209%
Did you know that Dream Card video poker games do not give the correct Dream Card in Bonus Poker when the four dealt cards are 5 singletons, with the lowest a four and the other three all less than a jack, with no straight or flush chance? The correct Dream Card would be the four, because of the premium win on a four fours. However, the Dream Card given will be one of the other ranks. What is the cost of this incorrect Dream Card, assuming the player always accepts it?
Yes, I was aware of this. For the benefit of the other readers, in Dream Card the game will sometimes give the optimal 5th card on the deal based on the first four randomly dealt cards. The probability of getting a Dream Card in Bonus Poker is 46.7%. The player can always reject the suggested Dream Card and switch to any other card still in the deck. However, the advice is always correct, as far as I know, except in this situation with Bonus Poker.
If the player gets the Dream Card, then the probability the other four cards will fall into this situation is 1.49%. Given the 46.7% probability of the Dream Card, this situation will happen with probability 0.70% or once every 144 hands.
Using my video poker hand analyzer, the expected value of a pair of fours in 8/5 Bonus Poker is 0.855134. The expected value of a pair of 5's to 10's is 0.813506. So the cost of the bug every time it occurs, assuming the player accepts the Dream Card, is 0.041628 in expected value.
The overall cost to the return of the game is 0.006955 × 0.041628 = 0.000290, or about 0.03%.
What is the probability of a royal flush if the player always drew to one? Assume if the player always chooses the suit with the most cards already to a royal.
The following table shows the probability of having 0 to 5 cards to a royal on the deal, assuming the player always choose the suit that already has the most cards to the royal, the probability of completing the royal, and the product.
Royal or Nothing Player
| Card to Royal on Deal |
Deal Probability |
Probability Complete Royal |
Product |
|---|---|---|---|
| 0 | 0.61538462 | 0.00000261 | 0.00000160 |
| 1 | 0.35444947 | 0.00003064 | 0.00001086 |
| 2 | 0.02835596 | 0.00070472 | 0.00001998 |
| 3 | 0.00173608 | 0.01057082 | 0.00001835 |
| 4 | 0.00007234 | 0.11627907 | 0.00000841 |
| 5 | 0.00000154 | 1.00000000 | 0.00000154 |
| Total | 1.00000000 | 0.00006075 |
The lower right cell shows a "royal or nothing" player has a probability of making a royal flush of 0.000006075, or 1 in 16,461.
What would be the probability of a royal flush in video poker if the player could peek ahead at the next cards on the draw?
Let's assume that the game picks five random cards for the draw, which will be waiting in a queue for the player to discard. For example, if the player discards three, he will get the next three in the queue. The answer is the same, by the way, if there is a specific card on the deal assigned to each card on the draw. That said, the following table shows the probability of holding each number to the royal on the deal, the probability of completing it on the draw, and the product. The lower right cell shows an overall probability of 0.00006075, which equals 1 in 16,461.
Perfect Peeker in Video Poker
| Card to Royal on Deal |
Deal Probability |
Probability Complete Royal |
Product |
|---|---|---|---|
| 0 | 0.61538462 | 0.00000261 | 0.00000160 |
| 1 | 0.35444947 | 0.00003064 | 0.00001086 |
| 2 | 0.02835596 | 0.00070472 | 0.00001998 |
| 3 | 0.00173608 | 0.01057082 | 0.00001835 |
| 4 | 0.00007234 | 0.11627907 | 0.00000841 |
| 5 | 0.00000154 | 1.00000000 | 0.00000154 |
| Total | 1.00000000 | 0.00006075 |
In your explanation of a video poker pull-tab machine, you give the example: "Even if the game looks like a five card draw video poker game your outcome is predestined. For example if you get a royal flush on the deal and throw all of it away you would get another royal flush on the draw." My question is, what happens if you throw-away cards that make a predestined outcome impossible (like a deuce in Deuces Wild for a predestined 4-Deuces or an Ace in a Double Bonus game for 4 Aces?) Maybe these types of games aren't offered but just those, like Jacks or Better, where no such situation is possible?
What I hear happens is a fairy comes along and changes your hand on the draw to whatever it was predestined to be. For example, if you were predestined to get two deuces on the deal and improve to four deuces after the draw, if you threw away the deuces, you would probably get the other two naturally on the draw, and then the fairy would change two junk cards to the two deuces you threw away.
In video poker, how often will a player have 0 up to 5 cards to a royal after the deal?
The answer is rather involved, because there are a number of ways the player can have a royal possibility, after the deal, in multiple suits. I assume the player always keeps the cards in the suit with the greatest chance at a royal and picks arbitrarily in the event two or more suits are tied for the most cards to a royal. That said, let me define some abbreviations:
- Royal cards = cards of rank 10 to ace.
- H = Royal cards in hearts.
- S = Royal cards in hearts.
- C = Royal cards in hearts.
- D = Royal cards in hearts.
- x = Non-royal card
The following table shows the number of combinations of every possible situation. A row will include all mathematically equivalent cases. For example, Hxxxx will include having one card to a royal only in any suit (not just hearts).
Combinations to Royal after Deal
| Hand | Cards to Royal | Combinations |
|---|---|---|
| HHHHH | 5 | 4 |
| HHHHS | 4 | 300 |
| HHHHx | 4 | 640 |
| HHHSS | 3 | 1,200 |
| HHHSC | 3 | 3,000 |
| HHHSx | 3 | 19,200 |
| HHHxx | 3 | 19,840 |
| HHSSC | 2 | 6,000 |
| HHSSx | 2 | 19,200 |
| HHSCD | 2 | 5,000 |
| HHSCx | 2 | 96,000 |
| HHSxx | 2 | 297,600 |
| HHxxx | 2 | 198,400 |
| HSCDx | 1 | 20,000 |
| HSCxx | 1 | 248,000 |
| HSxxx | 1 | 744,000 |
| Hxxxx | 1 | 719,200 |
| xxxxx | 0 | 201,376 |
| Total | 2,598,960 |
The next table shows the overall probability of having 0 to 5 cards to a royal after the deal.
Cards to Royal Probabilities
| Cards to Royal | Probability |
|---|---|
| 5 | 0.0002% |
| 4 | 0.0362% |
| 3 | 1.6637% |
| 2 | 23.9403% |
| 1 | 66.6113% |
| 0 | 7.7483% |
| Total | 100.0000% |
Not that you asked, but if a player followed a "royal or nothing" strategy, his probability of a royal per hand would be 1 in 23,162.
If I am using perfect betting strategy for video poker play, which game gives me the best chance of getting a royal flush?
As a basis of comparison, the probability of a royal flush in conventional video poker ranges from about 1 in 40,000 to 45,000, assuming optimal strategy. Here are some exact figures for some randomly selected games:
- 9-6 Jacks or better: 1 in 40,391
- 25-15-9-4-4-3 (Illinois) Deuces Wild — 1 in 43,423
- 9-7 Triple Double Bonus: 1 in 45,358
For Royal hungry players, the probability goes up significantly in Chase the Royal. This is an early video poker variant where the player may exchange a dealt pair of face cards for three to a royal flush on the deal. To make it a good value to trade, the game bumps up the win on the straight and flush, if you switch. The exact royal probability will depend on the base game and pay table. The probability is maximized with a base game of Triple Bonus and 8-5 pay table, at a royal frequency of 1 in 9,151. This includes both royals on the draw, which pay 800 for 1, at a frequency of 1 in 9,282 and on the deal, which pay 2000 for 1, at a frequency of 1 in 649,773.
However, it gets even better if you consider games in which the player must pay a fee equal to his base wager to enable a feature. In Draw Poker with Dream Card (not to be confused with Dream Card Poker), the player often gets the card of his dreams (assuming its a mathematician doing the dreaming) as the fifth card on the deal. The Dream Card probability of maximized in Jacks or Better, at 50.5%. In 9-6 Jacks or Better, the overall Royal frequency is 1 in 8,105. Keep in mind that with the fee to enable the feature, the effective win on a Royal drops to 400 for 1.
As long as I mention Dream Card Poker (a different game than Draw Poker with Dream Card), the Royal frequency in that game is not as high. It appears to be highest in 11-8-6 Jacks or Better at 1 in 15,034.
This answer does not consider Movin' On Up Poker, which is an old and obscure video poker game where the player gets two or three draws, instead of one. I don't know the Royal frequency in that game, but in Triple Draw, in which the player must pay a fee equal to five times his base wager to enable the extra two draws, I roughly estimate it to be about 1 in 4,000.
In conclusion, if you don't count games where the player must pay an extra fee to enable some kind of gimmick, my answer is Chase the Royal.
What is the cost in player errors if I play the optimal strategy for Not so Ugly Ducks in Illinois Deuces?
As a reminder, here are the pay tables mentioned:
Not so Ugly Ducks: 1-2-3-4-4-10-16-25-200-800.
Illinois Deuces: 1-2-3-4-4-9-15-25-200-800
Next, here is the return table for Not so Ugly Ducks, following the optimal strategy for that game.
Not so Ugly Ducks -- Correct Strategy
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Natural royal flush | 800 | 458,696,304 | 0.000023 | 0.018409 |
| Four deuces | 200 | 3,721,737,204 | 0.000187 | 0.037342 |
| Wild royal flush | 25 | 38,006,962,464 | 0.001907 | 0.047668 |
| Five of a kind | 16 | 61,961,233,656 | 0.003108 | 0.049735 |
| Straight flush | 10 | 102,392,435,976 | 0.005137 | 0.051368 |
| Four of a kind | 4 | 1,216,681,289,508 | 0.061038 | 0.244151 |
| Full house | 4 | 520,566,943,104 | 0.026116 | 0.104462 |
| Flush | 3 | 413,870,908,056 | 0.020763 | 0.062289 |
| Straight | 2 | 1,142,885,476,800 | 0.057336 | 0.114671 |
| Three of a kind | 1 | 5,325,911,611,716 | 0.267188 | 0.267188 |
| Nothing | 0 | 11,106,773,222,412 | 0.557199 | 0.000000 |
| Total | 19,933,230,517,200 | 1.000000 | 0.997283 |
Next, here is the return table for Illinois Deuces, using the correct strategy for that pay table. The lower right cell shows a return of 0.989131.
Illinois Deuces -- Correct Strategy
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Natural royal flush | 800 | 459,049,128 | 0.000023 | 0.018423 |
| Four deuces | 200 | 3,727,422,492 | 0.000187 | 0.037399 |
| Wild royal flush | 25 | 38,117,987,136 | 0.001912 | 0.047807 |
| Five of a kind | 15 | 62,201,557,608 | 0.003120 | 0.046807 |
| Straight flush | 9 | 98,365,859,016 | 0.004935 | 0.044413 |
| Four of a kind | 4 | 1,221,942,888,444 | 0.061302 | 0.245207 |
| Full house | 4 | 522,030,131,520 | 0.026189 | 0.104756 |
| Flush | 3 | 407,586,633,720 | 0.020448 | 0.061343 |
| Straight | 2 | 1,145,767,137,120 | 0.057480 | 0.114961 |
| Three of a kind | 1 | 5,342,397,992,292 | 0.268015 | 0.268015 |
| Nothing | 0 | 11,090,633,858,724 | 0.556389 | 0.000000 |
| Total | 19,933,230,517,200 | 1.000000 | 0.989131 |
The next table shows the return table using the combinations and probability from Not so Ugly Ducks on the pay table for Illinois Deuces. The lower right cell shows a return of 0.989131.
Illinois Deuces -- NSUD Strategy
| Event | Pays | Combinations | Probability | Return |
|---|---|---|---|---|
| Natural royal flush | 800 | 458,696,304 | 0.000023 | 0.018409 |
| Four deuces | 200 | 3,721,737,204 | 0.000187 | 0.037342 |
| Wild royal flush | 25 | 38,006,962,464 | 0.001907 | 0.047668 |
| Five of a kind | 15 | 61,961,233,656 | 0.003108 | 0.046627 |
| Straight flush | 9 | 102,392,435,976 | 0.005137 | 0.046231 |
| Four of a kind | 4 | 1,216,681,289,508 | 0.061038 | 0.244151 |
| Full house | 4 | 520,566,943,104 | 0.026116 | 0.104462 |
| Flush | 3 | 413,870,908,056 | 0.020763 | 0.062289 |
| Straight | 2 | 1,142,885,476,800 | 0.057336 | 0.114671 |
| Three of a kind | 1 | 5,325,911,611,716 | 0.267188 | 0.267188 |
| Nothing | 0 | 11,106,773,222,412 | 0.557199 | 0.000000 |
| Total | 19,933,230,517,200 | 1.000000 | 0.989038 |
The cost of errors is the optimal return for Illinois Deuces (second table) less the return for Illinois Deuces using NSUD strategy (third table) = 0.989131 - 0.989038 = 0.000093.
I read about the Reversible Royal game with the 105.22% return in your article at Wizard of Vegas. That return assumes optimal strategy, including for card order. What is the return if I assuming an average royal win? How about if I use ordinary 6-5 Bonus Poker strategy, which is the base pay table?
Assuming no strategy deviations, 1 in 60 royals will be sequential. The reversible royal jackpot pays 161,556 for 1. Any other royal pays 800 for 1. The average royal win is thus (1/60)*161,556 + (59/60)*800 + 17,396 for 1.
If we assuming all royals pay 17,396 and play optimal strategy based on that royal win, then the return drops to 103.56%.
If we play standard 6-5 Bonus Poker strategy, which is the base pay table, then the return drops further to 101.97%.
In your video poker programming tips, you explain how that although there are 2,598,960 possible starting hands in video poker, with a 52-card deck, there are only 134,459 classes of hands necessary to analyze.
My question is how many classes are there for joker poker?
For this one, I turned to my esteemed colleague, Gary Koehler, who is an expert at video poker math. Here are his answers, according to the number of jokers:
- 1 Joker: 150,891
- 2 Jokers: 169,078
- 3 Jokers: 189,189
- 4 Jokers: 211,406
- 5 Jokers: 235,925
In your video poker programming tips, you explain how that although there are 2,598,960 possible starting hands in video poker, with a 52-card deck, there are only 134,459 classes of hands necessary to analyze. My question is if someone were playing a game where the order of the cards matter, like Ace$ Bonus Poker or one with a jackpot for a sequential royal, how many different classes of hands would be needed to analyze?
For this one, I turned to my esteemed colleague, Gary Koehler, who is an expert at video poker math. His answer is 15,019,680.
What is the average number of cards held after the draw in video poker?
The following table shows the average number of cards held in 10 different games and pay tables. The average of the games listed is 2.05.
Average Cards Held in Video Poker
| Game | Pay Table | Return | Ave. Cards Held |
|---|---|---|---|
| Bonus Deuces | 10-4-3-3 | 97.36% | 1.845550 |
| Deuces Wild | 25-15-9-5-3 | 100.76% | 1.926010 |
| White Hot Aces | 9-5 | 99.57% | 2.055630 |
| Super Double Double Bonud | 7-5 | 99.17% | 2.057280 |
| Double Double Bonus | 9-5 | 97.87% | 2.058390 |
| Triple Double Bonus | 8-5 | 95.97% | 2.072620 |
| Bonus Poker | 8-5 | 99.17% | 2.080610 |
| Jacks or Better | 9-5 | 98.45% | 2.081030 |
| Bonus Poker Deluxe | 8-5 | 97.40% | 2.150470 |
| Double Bonus | 9-6-5 | 97.81% | 2.173550 |
I'm aware that if you win $1,200 or more on a machine you have the option to be paid by check. That said, let's say, for the sake of argument, that I wish to convert cash to checks issued by a casino. Please assume I play 9/6 Jacks or Better perfectly at a $25 denomination (or $125 total bet). How much of my cash could I expect to lose converting it to checks?
For 9-6 Jacks or Better you will win $1200 or more with every four of a kind or higher. The return for a full house and less is 0.911103. You'll have to churn though your original bankroll 1/(1-0.911103) = 11.249016 times until your cash has either been converted to check or lost to the house edge. The house edge is 0.004561. Thus, you can expect to lose 0.004561 × 11.249016 = 0.051306 times your original bankroll.
Interestingly, the cost to convert cash to checks is less in 9-6 Double Double Bonus, despite the higher house edge. That game has higher wins for all four of a kinds, so your checks are larger. In that game, the return from a full house and less is 0.777138. That means, you'll have to cycle through your bankroll 1/(1-0.777138) = 4.487076 times to convert it to check. The house edge in 9-6 double double bonus is 0.010192. Thus, the expected loss converting to cash is 4.487076 × 0.010192 = 0.045733.
You could cut down the cost even more with strategy deviations that target four of a kinds and higher, but I'll leave that as an exercise for the reader.
This question is asked and discussed in my forum at Wizard of Vegas.