Deal or No Deal (Cruise Ship Version)
On September 29, 2023, aboard the cruise ship Celebrity Reflection, I attended a Deal or No Deal game show. I took pictures and saved one of my cards to analyze when I got back home. This newsletter shall be that analysis.
On the day of game, cards cost $15 each. However, you could purchase four for $50, for an average of $12.50 per card, a small economy of scale. I know there was some incentive to buy cards long before the day of embarkation, but I don’t recall the details. Without knowing the value of the game, but figuring it was probably a very negative expected value, I didn’t buy any cards until the day of the game. My motive for buying cards the day of the game was largely the entertainment value.
The game was divided into two sessions. In each session a card was randomly and electronically picked from all those in play. The card holder was then invited to the stage to play.

This above image shows cruise director Angela and the lucky player of game 1.
The game on the ship played just like that on the television show, except there were 20 cases instead of 26 and the prizes were lower. Banker offers were about 90% of the average of the unopened cases.
There actually was a veiled banker, who I recognized as another member of the staff who sometimes filled in for Angela in her duties as cruise director.
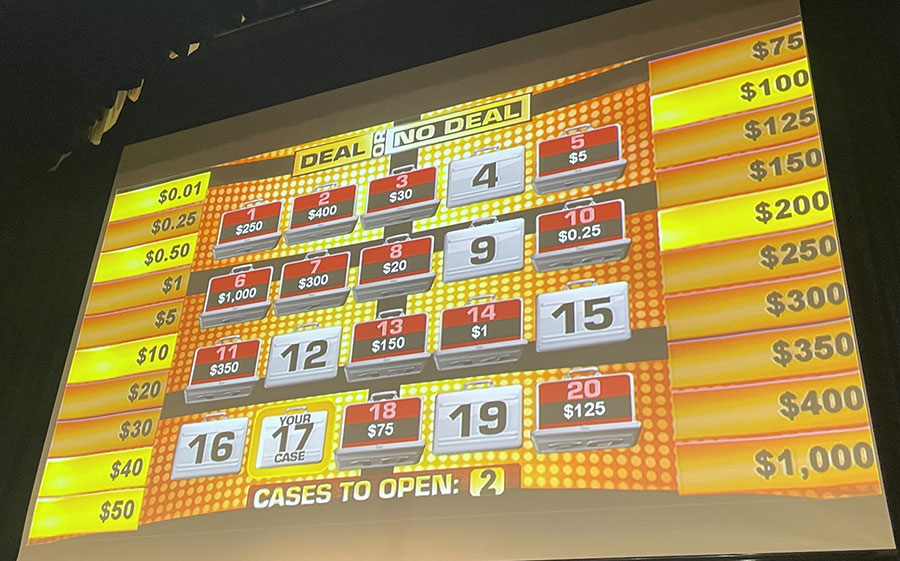
The picture above shows the game mid-state. The lit amounts on the left and right show those still in play. The rest were in already opened cases.
After the traditional game was played, the same player got a chance at the Banker’s Wheel. I was told this was a fair wheel, with a 50% chance at a free cruise. The other 50% were casino match play chips of $25, $50, $100 or $250. They did not state anything on the type or value of the “free cruise.” The rules on the back said, “If the free Cruise is offered as a prize, the itinerary, length and accommodation is determined by the Cruise line and does not include air, transfers, hotel, taxes and gratuities, or any other fees applicable to the cruise.” Having won or earned four cruises in Las Vegas, I can say that they are generally the lowest level cabin and they blackout all the best dates.

The image above shows the Banker’s Wheel.
Finally, every card had a chance to win consolation prizes. These cards had 20 cases with the same prizes as used in the game, which were randomly shuffled behind the flaps on the card. Players in the audience didn’t have to worry about opening the flaps and keeping score as the computer knew the location of every prize on every card and who owned every card. After each set of case openings, the screen showed the players in strong contention for any prizes.

The image above shows the consolation prizes according to the number of matches between the player’s card and the location of the prizes in the game. In this update, partially through a game, 50 cards had one match and 15 had two.

The image above is of a typical card. Each case had a flap revealing one of the 20 possible prizes.
My analysis of the game depends on some information I don’t know, in particular the number of competing cards and the value of the cruise.
It is easy to say that the average of the cases is $155.33. This is the value of the base game, assuming the player never accepts a below-value banker offer. I roughly estimate there were 300 cards in play. That would give each card a value per game of $155.30/300 = 4.14 cents.
To analyze the Banker’s Wheel, we need to know the value of the free cruise. If we generously put the free cruise value at $2,000 and assume full value of the casino match play, then the value of that element of each game is $1,053.13, which is significantly more than the base game. If we divide that by the 300 estimated cards in play, the value of each card per game is 28.08 cents.
Analyzing the consolation prizes for matching prizes on the play cards is a famous problem in mathematics, usually known as the Secret Santa problem. It stems of the office game where everybody in the office is assigned a random person to give a gift to. The way the question is usually stated is what is the probability that any specified number of people draw their own name. In this case, we have to analyze the probability of 2 to 20 matches.
The exact probabilities are beyond the scope of this newsletter. Perhaps I’ll write another newsletter in the future about that (but probably won’t). For now, we will have to go with estimates, which should be very close. If you are interested in the exact math, it has been discussed in my forum at Wizard of Vegas
The following table shows my estimate probability of 2 or more matches, the associated prize, and the expected win for each possible outcome. The lower right cell shows an expected win of $3.20 per card per game.
| Matches | Probability | Win | Return |
| 8 or more | 0.000010 | $ 5,000 | $ 0.05 |
| 7 | 0.000073 | $ 500 | $ 0.04 |
| 6 | 0.000511 | $ 400 | $ 0.20 |
| 5 | 0.003066 | $ 200 | $ 0.61 |
| 4 | 0.015328 | $ 50 | $ 0.77 |
| 3 | 0.061313 | $ 10 | $ 0.61 |
| 2 | 0.183940 | $ 5 | $ 0.92 |
| 1 | 0.367879 | $ - | $ - |
| 0 | 0.367879 | $ - | $ - |
| Total | 1.000000 | $ 3.20 |
To review, I get a value of $0.04 for the base game, $0.28 for the Dealer’s Wheel and $3.53 for the consolation prizes, for a total of $3.53. Recall they played through two games, so double the total expected win to $7.06. At a cost of $12.50 per card (buying four for $50), the expected return is 56.48%. In other words, a house edge of 43.52%. If there were any no-shows, it would increase the value. However, with the vast majority of the value in the consolation prizes, the number of cards in play doesn’t really matter very much in terms of the game value.
It should be noted that Angela said they wouldn’t credit any free play until some hours after the show and to check with the concierge desk about it. I doubt many people did, as this was the last full day of the cruise. I never did check if I won any free play, although my name was not listed as being in contention towards the end of either game.
I would also like to note that the same person was called to play both games! The second time, he sent his wife up. I later asked him how many cards he had in play, to which he answered five.
Overall, the game was very well done. The hostess, Angela, did an outstanding job. I had a fun time as I think Mrs. Wizard and my friend who I cruised with did as well. Would I play this game a second time? Probably not, but as a fan of game shows, it’s worth doing once despite the game offering about the same value as a lottery ticket.

The image above shows the full rules.
For more information, visit Timeplay.com, the makers of this and other multi-player games for cruise ships.




