Ask The Wizard #265

You’re confusing the mean and the median. Let’s look at my situation as an example. I’m a 45-year-old male. My life expectancy is 78.11 years, yet I have a 50.04% chance of making it to age 80.

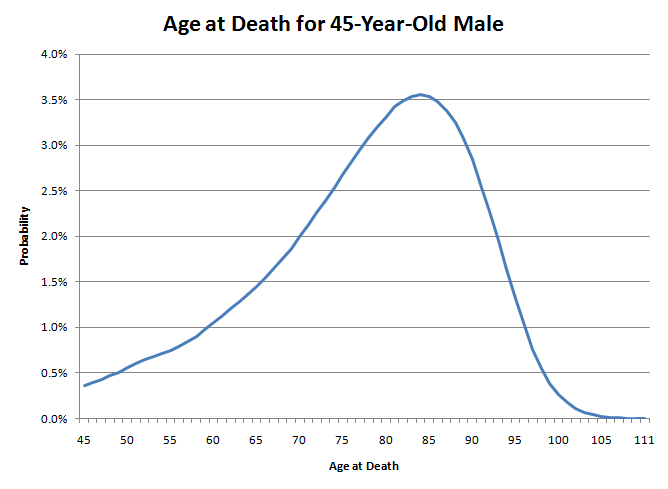
My age at death will be like throwing a dart at this graph. Notice how the left tail is a lot fatter than the right. That means my probability of death right now is quite low. However, as I get older, the probability of death in the next year will keep getting higher. For example, for a 45-year-old male the probability of surviving to 46 is quite high at 99.64%. However, at age 85 the probability of making it to 86 is 89.21% only. It is like nature slowly pushing a knife in your back. At first it probably won’t kill you, but with each passing year, the odds slowly increase that it will. However, once you get to the late seventies nature says enough with the games and really starts shoving it in.

So if a lot of 45-year-old men throw darts at this graph, 49.96% will hit between 45 and 79, and 50.04% will hit between 80 and 111. However, the lucky half who make it on the right side of the graph will probably not live much past 80. Once a male reaches 80 he can expect to live 7.78 more years only. Meanwhile, many in the unlucky half who don’t make it to 80 will die much younger than that. So it is the many young deaths that pull down average life expectancy.

For a similar situation, consider a die numbered 10, 20, 30, 31, 32, 33. The average is 26, yet there is a 2/3 chance of rolling more than that.

As an example of how the mean and median are different, suppose we add two more deaths to the sample. One death at 46 and one at 81. The probability of making it to 80 doesn’t change, but the average life expectancy at age 45 would go down.

This question was raised and discussed in the forum of my companion site Wizard of Vegas.

Good question. The house way says to split up five aces, playing two aces in the low hand. The exception is to retain the five of a kind in the high hand if you can play two kings in the low. My pai gow poker page indicates seven house ways from Las Vegas and Atlantic City, and all of them include this exception. The three from outside those two cities do not.

The probability of getting four aces, the joker, and two kings is 1 in 25,690,513. Assuming the dealer is banking, the only situation where this exception would help is if the player had a four of a kind or better in the high hand. The probability of that is about 1 in 300. The probability of both happening is about 1 in 7.6 billion.

According to the Nevada Gaming Control Board, there were 306 pai gow poker tables in Nevada in 2009. If we generously assume 60 hands per hour, two players per table, and every table is open 24/7, then it would take 23.7 years for this exception to occur and make a difference in the outcome.

So the casinos are requiring every single pai gow poker dealer to memorize this exception when it may never have made a difference in the outcome in the history of the game. I speculate the same person who thought of adding that rule was the same person who decided that the A2345 straight, known as "the wheel," is the second highest straight.

That is a classic problem in the history of the field of probability. Many people incorrectly think the answer is 18, because the probability of a 12 is 1 in 36, and 18×(1/36)=50%. However, by that logic, the probability of getting a 12 in 36 rolls would be 100%, which clearly it isn’t. Here is the correct solution. Let r be the number of rolls. The probability a roll isn’t a 12 is 35/36. The probability there are 0 12s in r rolls is (35/36)^r. So we need to solve for r in the following equation:

(35/36)^r = 0.5
log(35/36)^r = log(0.5)
r × log(35/36) = log(0.5)
r = log(0.5)/log(35/36)
r = 24.6051

So there isn’t a round answer. The probability of rolling a 12 in 24 rolls is 1-(35/36)²⁴ = 49.14%. The probability of rolling a 12 in 25 rolls is 1-(35/36)²⁵ = 50.55%.

If you want to make a bet on this, say you can roll a 12 in 25 rolls, or somebody else can’t in 24 rolls. Either way you’ll have an advantage at even money.

I would lay 10 to 1 you'll get paid, although they may have a few words with you first. The reason I have some doubt is a sports book can rescind a wager that was obviously made in error, with permission of the Gaming Control Board.

"A book may not unilaterally rescind any wager without the prior written approval of the chairman." -- Nevada Regulation 22.115

While this right exists, based on anecdotal evidence, it is very seldom invoked. Let me know what happens.

p.s. I was notified later by the questioner that he was paid without much trouble.

Yes, it will, after e^100,000 -1 seconds. See my mathproblems.info site, problem 206, for two solutions.

This question was raised and discussed in the forum of my companion site Wizard of Vegas.